Anyone please provide a brief solution or explanation for this problem. I found this quite difficult.
To represent the change of coordinates in the rotating frame of reference, we can take one point with the coordinate system for clarity. Let’s take any point and see that its x and y coordinates will constantly change from angular velocity, and the third coordinate does not change, which as I understand the rotating system is rotating along the z-axis.
(sorry, I will send later)
Then we get equations:
The position vector of the particle in S is \vec r=x\hat x+y\hat y+z\hat z. It gives us coordinate of rotation system.
If we consider a point from a rotating reference frame, it is not difficult to guess that it is a non-inertial reference frame. So the point will experience the Coriolis force and the centrifugal force.
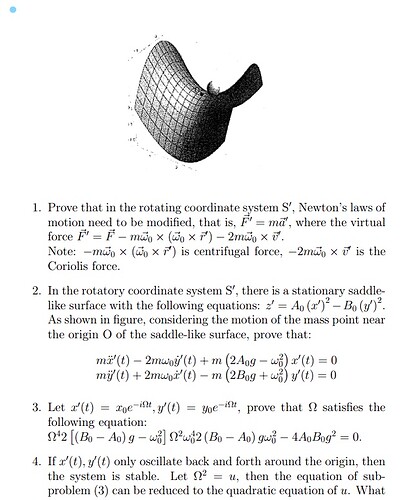
Moving on to more complicated things. We are given that there is a stationary saddle-like surface in the rotating report system, which is indicated in the figure.(I think it would be better, if you read about that in Internet.) Having the formula for z, we find r and the open equation for all forces.
When we consider a rotating frame of reference, we must not forget about oscillations. They should be written as, where i is a complex number, and the angle respectively equals the rotation of the system:
Сonstant A is equal to the amplitude of the system(x_0):
Ah, thanks for this… however I am already well versed with this concept, but I don’t understand how to apply this to the saddle surface etc.
I studied this concept from the book Introduction to Classical Mechanics, David Morin (red cover) but still I think I’m not at the level to apply this to different or more complex coordinate systems.
(maybe this will help you to understand what’s going on in the rotating system)
I found some resourses about this
Oh thanks a lot! I’ll take a look at these vids.
By the way, there are other problems which I would like to contribute to the community. There are some problems which I have translated from various countries. Where can I submit them? @Anton
You’ve translated them to English from some other languages? You have them in PDF format?
I have translated them into English. The problems are from the IPHO selection exams of Russia, Vietnam, China, Taiwan etc. They are not publicly available. They are in PDF format in latex.
you can send an archive to @Zhabka
he’ll upload it to olympiads.bc-pf.org
Ok I’ll email it, if it is alright!
- To answer the first question you can firstly take a look at my post here:
The radius vector in rotating system of coordinate S’:
Then you need to take into account the fact that: (its obvious)
Firstly take the first derivative:
Then take the second derivative:
You should use these equations:
and take their derivatives
By the way, formulas of the unit vectors \vec{\hat x'}, \vec{\hat y'} are similar to the ones, which were in polar coordinates:
(You’re able to finish it)
2. Answer to the 2nd question is simple. You need to use the equation:
Consider the projections of the force on axes x and y:
You also should use the equation of surface:
to find an expression for potential energy:
The force vector is an anti-gradient of potential energy:
Using this and also completing some of the cross-products you will solve the problem
(You’re able to finish it)
3. You shouldn’t have any problems answering this question, because you just need to put the x’,y’ functions to the differential equations from question 2:
(You’re able to finish it)
I think that you’re capable of solving the 4th and 5th problems, but take into account that in a stable state, where oscillation occurs only around the origin you can use these kinds of approximations for displacements:
and also the force must be equal to zero in a stable equilibrium
Thank you!
You just need to know the basic concepts. Read it again and solve the problems one more time if you feel like you didn’t really understand it