Let’s tackle the problem step by step as quoted by Alibi. The task is to:
-
Determine the radius of a platinum atom.
-
Calculate the number of active centers on the surface of a single catalyst particle.
-
Determine how many CO2 molecules adsorb on the surface of a single particle at a given pressure and Langmuir constant.
First, we need to find the radius of a platinum atom. Platinum has a face-centered cubic (FCC) lattice, where the atoms touch each other along the face diagonal. The face diagonal in an FCC unit cell is equal to four times the atomic radius (4r), and it can be related to the lattice parameter (a) by the equation \sqrt{2}a = 4r. Given that the lattice parameter a = 0.392 nm, we can calculate the atomic radius r.
Solve for r:
r = \frac{\sqrt{2}a}{4} = \frac{\sqrt{2} \times 0.392 \text{ nm}}{4}
Now calculate r:
r = \frac{\sqrt{2} \times 0.392 \text{ nm}}{4} = \frac{1.414 \times 0.392 \text{ nm}}{4} \approx 0.139 \text{ nm}
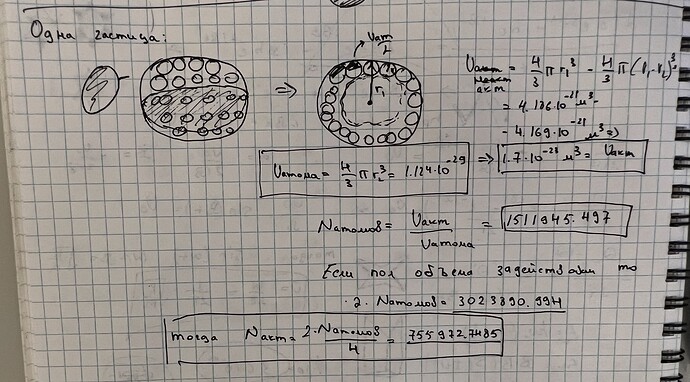
Next, we need to calculate the number of active centers on the surface of a platinum particle. An active center consists of four atoms. Since the particle is spherical, we first find its surface area and then determine how many groups of four atoms fit on this surface.
Given that the diameter of the particle is 0.2 μm (0.2 \times 10^{-6} m), the radius of the particle (R) is half of that:
R = 0.1 \times 10^{-6} \text{ m} = 100 \text{ nm}
The surface area of a sphere (A) is given by A = 4\pi R^2:
A = 4\pi (100 \text{ nm})^2 = 4\pi \times 10^4 \text{ nm}^2
Now, we must calculate the area occupied by four atoms on the surface. Since we are dealing with an FCC lattice, the surface atoms are arranged in a square pattern. Therefore, the area occupied by one atom is a^2/4, and by four atoms (one active center) is a^2 because there are four atoms in each face-centered cubic unit cell on a square face.
\text{Area of one active center} = a^2 = (0.392 \text{ nm})^2
To find the number of active centers (N_{\text{active}}), divide the total surface area of the particle by the area of one active center:
N_{\text{active}} = \frac{A}{a^2}
N_{\text{active}} = \frac{4\pi \times 10^4 \text{ nm}^2}{(0.392 \text{ nm})^2}
Calculate N_{\text{active}}:
N_{\text{active}} = \frac{4\pi \times 10^4}{0.153664} \approx 814,000
Lastly, to find out how many CO2 molecules adsorb on the surface, we use the Langmuir adsorption isotherm model. This model suggests that for each active site, there can be at most one molecule adsorbed. However, not all sites will have adsorbed molecules due to the dynamic equilibrium between adsorption and desorption. The Langmuir isotherm is given by:
\theta = \frac{K P}{1 + K P}
where \theta is the fraction of the surface covered by the adsorbed molecules, K is the Langmuir constant, and P is the pressure of CO2 (in Torr). Given K = 2.5 \times 10^{-3} \text{ Torr}^{-1} and P_{\text{CO2}} = 200 \text{ Torr}, we can calculate \theta:
\theta = \frac{(2.5 \times 10^{-3} \text{ Torr}^{-1}) \times 200 \text{ Torr}}{1 + (2.5 \times 10^{-3} \text{ Torr}^{-1}) \times 200 \text{ Torr}}
Now calculate \theta: